40 years of observations of the Audouin’s Gull (Ichthyaetus audouinii) population by Dr. Meritxell Genovart and Dr. Daniel Oro of the Centre d’Estudis Avançats de Blanes (CEAB-CSIC) have made possible to model, for the first time, a collapse of a population with dispersion by social copying after a disturbance in the ecosystem.
The mathematical model developed by Prof. Lluís Alsedà and Dr. Josep Sardanyés of the Center de Recerca Matemàtica (CRM) in collaboration with the scientific team of CEAB-CSIC and Prof. Alan Hastings of the University of California Davis has allowed to reproduce the dynamics of the escape and dispersal of the Audouin’s Gull population at Punta de la Banya (Banya Cape) in Delta de l’Ebre. This strong dispersal was due to the arrival of few predators at the area, mainly foxes.
The mathematical model, which has been adjusted to the field data using artificial intelligence (AI) algorithms, shows that the main dispersal mechanism of the species is social copying, which could be extrapolated to the study of other social species.
First dataset since the settlement of the Audouin’s gull population
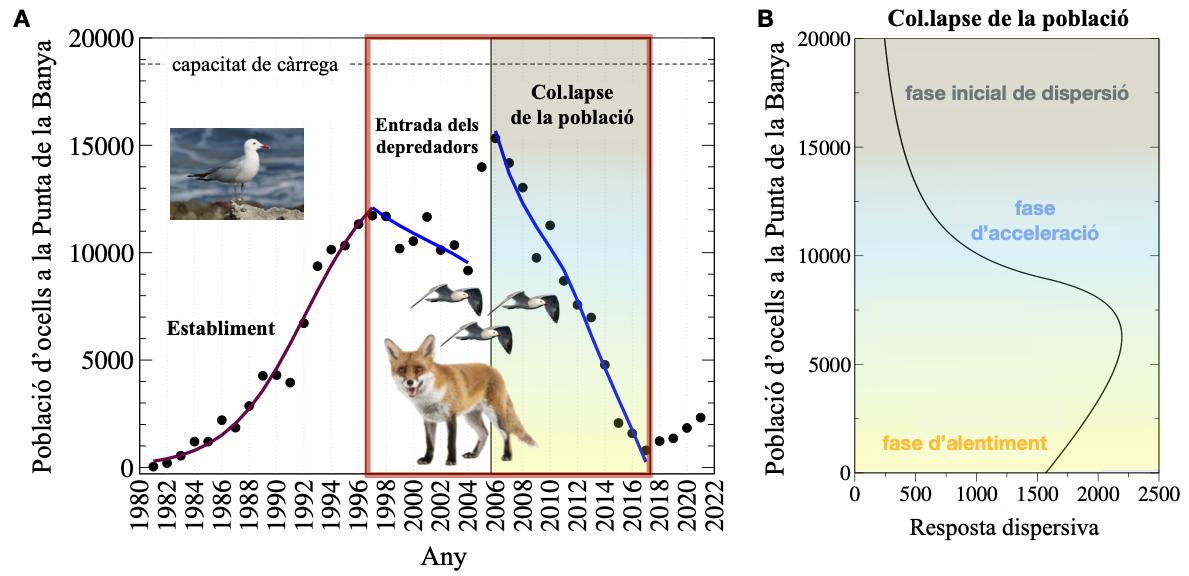
Since 1981, a CEAB-CSIC team in collaboration with the Natural Park of Delta de l’Ebre have performed an exhaustive monitoring of the number of breeding Audouin’s Gulls in this protected area. These data are very valuable since the population has been monitored since the initial establishment of the first individuals, which rapidly became the largest colony in the world, finally collapsing in only twelve years. Punta de la Banya has been a key breeding site to study the metapopulation dynamics of the species throughout the western Mediterranean, which includes 90% of the world’s population of the species. A metapopulation consists of different spatially separated subpopulations of a given species which are connected by migration/dispersal processes.
During the initial phase, from 1981 to 1997, the gulls found such good breeding conditions and the population growth was exponential, thanks to very high fertility and immigration rates never recorded before, and the colony contained up to 73% of the population, something absolutely unheard of.

The second phase began with the arrival of foxes to the colony, which were attracted by the great availability of colonial birds breeding in Punta de la Banya, generating a disturbance that progressively eroded the quality of the habitat. This colonial bird has no active defense mechanism against terrestrial predators and their only response is to disperse to other breeding areas. The gulls continued to breed for a few years waiting for the situation to get reversed and in the meantime, they gathered information about other places to migrate and breed.
After a few years of steadily worsening breeding conditions, a cascading emigration occurred ending in the collapse of the population, which in a few years became only 3% of the world’s population.
A unique mathematical model describing the population dynamics of Audouin’s Gulls
Prof. Luis Alsedà and Dr. Josep Sardanyés, together with Prof. Daniel Oro, have developed a mathematical model to describe the dynamics of the gull population. Using field data collected by the CEAB-CSIC scientific team, they were able to study the initial exponential growth of the population and find the most relevant ecological parameters accountable for the dynamics observed in Punta de la Banya, estimating the rates of reproduction, immigration, and competition (for food and/or nesting areas) between members of the same population.
The mathematical model has allowed to estimate the carrying capacity of the Punta de la Banya ecosystem (how good is the study site to host the bird population due to food availability, environmental conditions, and space) as well as the expected population equilibrium.
With the arrival of predators some gulls began to disperse before reaching the population equilibrium predicted by the mathematical model. And this is where the novelty of the model appears. The team has been able to demonstrate that by modeling different dispersion terms and applying AI methods such as genetic algorithms, that population dispersal after disturbance responds to the phenomenon of social copying.
“Organisms need to store information to reduce the uncertainty inherent in their environment,” explains Prof. Daniel Oro. “This information is aimed at optimizing the search for resources, such as food or shelter from predators. However, social animals have their own mechanism shaped by evolution that is so extraordinarily simple and effective that it has appeared from bacteria to large vertebrates, including our species: social copying. When we face a threat and we don’t have the information we need to make a decision, what we do is copy what others do.”
The study describes and quantifies, for the first time, a social tipping point
Nowadays, we often talk of tipping points linked to ecosystems: such points define critical boundaries beyond which dynamics undergo major changes that can lead to the extinction of species and loss of biodiversity. These regime shifts, which have been identified in many theoretical studies and real systems, are often irreversible.
This study presents a groundbreaking scientific result: the characterization and quantification of social tipping point, in this case related to bird dispersal as a function of population size. This social tipping point implies that, when a given population threshold is achieved due to dispersal, the rest of the population copy this behavior as in an avalanche. This abrupt response in dispersal, which has been shown to be highly nonlinear, caused the collapse of the colony in only 12 years.
Dr. Sardanyés explains that “Thanks to the mathematical model we have been able to estimate the ecological parameters of the population and demonstrate that the most important mechanism in the escape of gulls between 2006 and 2017 was that of social copying. Furthermore, the model has allowed, for the first time, to quantify a social tipping point in the context of metapopulations, which is a very important ecological concept that has implications for species persistence and environmental management. Until now, most metapopulation models assumed that dispersal was exponential and increased with population size. We have determined that for some species this no needs to be the case. Thus, our work provides valuable information for the future modeling metapopulation dynamics for social species showing this type of behavior“.
In a context of global change, understanding the collapse of populations is fundamental
The results of this research could also be generalized to study disturbance processes in stable ecosystems and social species responses to external perturbations.
“This type of process closely resembles what is recorded in human populations subjected to a severe perturbation over time, such as war conflicts,” says Prof. Daniel Oro, “and highlights the significance of a behavior as simple as social copying in unexpected population responses, such as collapses.”
The study shows the role of self-organized collective behavior in population dynamics and in social species population collapse.
“This work is the result of an ecologist’s (Daniel Oro) intuition about the importance of social copying in the dispersal of a colonial species,” explains Josep Sardanyés, co-author of the paper. “And it is also an example of interdisciplinary work, with elements of ecology, statistics, artificial intelligence, dynamical systems and mathematical modeling. The characterization and quantification of the response in dispersion as a function of population size, which we have seen is highly nonlinear and varies in a non-trivial way, could not have been obtained without using mathematical modeling.“
Reference article:
Oro, Ll. Alsedà, A. Hastings, M. Genovart, J. Sardanyés. Social copying drives a tipping point for nonlinear population collapse. Proceedings of the National Academy of Sciences USA 120(11), e2214055120 (2023) doi:10.1073/pnas.2214055120


